In following files some problematic issues concerning the dimension degree step 4 → 3 are discussed.

Headlines - different aspects:

I. Introduction
II.  Rotation
III. Geometrical aspects
IV. Multiplicity of Mass and its Distribution
V.  Mass as property - once again
VI. Step 4 → 3 in terms of Forces

Introduction:

The start of Physics:
Physics starts at the border to metaphysics, or rather at the triple-point between metaphysics - mathematics/geometry - and human concepts expressed in words.

The start of Universe:
Universe is proposed to be a "blown up singularity" as in this model and there it starts. (But it doesn't seem to be any consensus about the size of this "singularity.)
   Inflationary or not, the Big Bang could perhaps be described in terms of "osmosis" (!), a scalar field of density, the derivation of which is vector fields.

Compared with the model here, sketched in Presentation, scientists seem to look at the creation of Universe backwards, from the end of the 5-dimensional chain: the first stage after Big Bang is described as a Universe of "radiation" only. Probably electromagnetic (EM) waves (?), referring to the observed nearly homogeneous background microwave radiation. This radiation should then in some way partly transform to electrons, protons and atoms.
   At the same time they imagine all forces united at start and gravitation as the first one being "precipitated" from the other ones. And, according to other books, the only way one have observed "matter" created by EM-radiation is when energy rich photons create pairs of electrons/positrons (e-/e+), in the neighborhood of heavier masses or atoms (as a condition).
   It's not easy to unite these different aspects in terms of established scientific data.

According to the 5-dimensional chain of concepts in the model here, reading it outwards towards lower dimension degrees:

5 →4 →3 →2 →1 →0/00,

forces or vector fields as Acceleration/Gravitation, FA/ FG come first, in next step the EM-force, and the polarization Mass — Space before Matter with polarization of Charge, and waves in steps → 2 →1→0/00.
    However, reading the chain as from a perpendicular viewpoint, with debranched degrees in first steps meeting the other way around, illustrated in the figure below, the different proposals of the scientists seem more easily understandable.

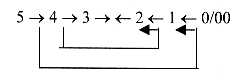
What they imagine as purely radiation becomes in this interpretation only the debranched degrees from first steps, but should be complemented with the structures from vector fields in steps 4 →3 →(2).

It's very much that physicists don't know:
They don't know what Gravitation is and how it can act over distances, just imagine a lot of small quanta as gravitons gathering to a discontinuous "field" or the inverse, a field quantified in those imagined quanta.
   Some believe they can skip gravitation and replace it with the curvature of space - but only half of the curved way of light around the sun could be explained by the curved space, the other half through "gravitation".
   And they don't seem to know why big masses are curving the space around them.
   They don't know what Mass is, what is giving matter the property of Mass, but particle-hunting physicists now are looking for the "Higgs' particle" that should give this mass property.
   They didn't know, at least forty years ago, what Charge is and probably not yet.
   They don't know what "Spin" is, an invented property, it's neither this, nor that - but still useful.
   Not to mention quantum mechanics, which they readily admit they don't understand but still have rules and some words and mathematics to manage.

Yet, these concepts, forces, mass, charge and spin are the main properties which they use in the "standard model" to describe the realities, besides time and distances.

Such questions, what something "is", are by definition, hard to answer: The answers have to be given in any of the three areas meeting at the triple-point. Words and mathematics for the physicists' part. And there are a lot of concepts and different mathematics to travel around in. Concepts have to be defined through other concepts and these through others again.
   A force, for instance, was earlier defined through its effect, as something that changes a motion, its direction or speed. Now, in the "standard model", forces are described similar to human talk: two persons as "particles" throwing words as small field quanta, photons or other things, on each other. Something like bacteria, joining to exchange parts of their genetic code, just to describe it on the superposed level of living societies.
   One conclusion: If we want an understandable description in words, it seems as we have rather much of a free choice when it comes to which concepts to use.

The concept "Dimension":
A special problem is the different ways to define the concept "Dimension".
   In this model we have defined an outward or inward directed vector field as 4-dimensional. In which sense isn't it 3-dimensional - or representing an infinity of dimensions as extensions in an infinity of directions?

- Physicists want one dimension for each independent variable. Independent? Shall we believe that? According to the model here nothing is really independent, at bottom. This "independence" seems only to refer to elementary geometry and the usual coordinate system and relations where the scalar product of vectors are zero.

- Mathematicians as Hilbert creates an infinity-dimensional room for possible "states"...

- In ordinary speech of today there is often talk about this and that as another dimension, usually referring to another aspect, characterized through some kind of contrast or opposition.

- In elementary geometry we have the usual coordinate system of 3 axes and they are indeed also characterized by opposite directions from the origin, with signs plus and minus. One says that the 3 axes are needed to completely define the position of a point in space. However, this view disregards identification of the origin, where the axes cross each other, and the "directions", the "signs" (+/-).

   One definition concerns extension, actually built on the basic concept Distance. A point has no extension, represents dimension 0. The extensions are created first with distances and space.

To this comes the little problem with a curved line or surface: a curved line is 1-dimensional in itself but needs 2 dimensions, a curved surface 3 dimensions for its existence. What about a curved space - ? - or a "curved" mass? * And in the String theory a linear string needs 10 more dimensions plus Time for its vibrations to express such things as mass and charge and what else: still another definition of "Dimension" as it seems.
   *A point has in these days been defined as something with an infinite    radius of curvature - a really self-absorbed definition!

So, in which sense do we use the fundamental concept Dimension in this model, when describing vector fields as 4-dimensional in relation to Masses and Vacant Space as 3-dimensional? The infinity of directions outwards from a point or inwards towards the point is obviously not seen as different dimensions, just as a property in this dimension degree (d-degree).
   When talking about 4 dimensions we use the kind of definition which concerns how many data that are needed to identify a certain unit (3 for position in space, + 1 for directions outwards/inwards. When talking about Mass and Space as 3-dimensional in relation to vector fields, we still keep to the polarization concept of two complementary "structures" but suddenly also enter into the definition of dimensions as extensions, viewing Masses and Space very elementary, without complex curvatures, only in their external form.
   Do we use the same definition of dimensions in these descriptions? What happens - in the "degradation" of our viewpoint - or physically in the formation of enclosed volumes as masses - when stepping from 4 to 3 dimensions?
   How does an infinity of directions (vdiv / vcon) transform to an ordinary 3-dimensional form in the step 4 →3 according to the model here? In which sense could Mass be interpreted as 3-dimensional in relation to vector fields as 4-dimensional?

There are many questions connected with this one:
- Why do all masses of universe rotate?
- Why this manifolds of masses and unity of Space in cosmos?
- And again: What is Mass, how should Mass be interpreted as created through this step 4 →3 →(2)?

See also a new suggestion about "GA-waves".

Rotation

Why do all celestial masses rotate, cosmic clouds and elementary particles, even haploid eggs in the oviduct? It's an obvious reality for celestial bodies in macrocosm and in microcosm, but what is the best way to explain it?
   In which sense do principally anti-parallel vectors (outwards-inwards) towards a center, in step 4→3, change to perpendicular ones, an angular step proposed in the model here? And what makes expansion-contraction transform to rotational phenomena? It's difficult to find any convincing explanation in the used literature behind this discussion. The question is connected too with the problematic turbulence.

1. Rotation as a 2-dimensional motion:
1a) In terms of the elementary 5-dimensional conceptual structure of our model:
   We may ask: What is lost in the dimension step 4→3? D-degree 4 is defined as vector fields, and a vector, according to the established definition, is a physical quantity that besides numerical values must be given a direction. So it has to be the character of Direction that is lost in the structure in this step - and translated to motion. A circular form has no direction in the sense of inwards - outwards.

1b) In d-degree 3 two degrees should be viewed as debranched and transformed to external motions. A 2-dimensional motion is rotation. (See files Presentation and Motion.)* Hence, rotation is viewed as one expression for the polarization of Direction in "poles 4a — 4b
   There is also the general view on a dimension chain as steps towards a more and more specified (crystallized) direction towards "one-way" character. (From a chapter on "chance", not yet on this site.)
   The one-way direction in rotation could be a Testimony of the rightness in the view of masses as one "pole" or partial structure in relation to a complementary one, the Vacant Space. (It may demand an excuse pointing out the natural fact that Space "rotate" in the complementary direction around celestial bodies.)

* Now it seems wrong to state that a celestial body has only motions in 2 dimensions. A planet like the Earth has its translation too, its pathway motion (and a slow rotation of its rotational axis!). How then justify the proposal? We could perhaps presume that it is the orbital as a 2-dimensional plane of each planet that moves around the sun, not the 3-dimensional planet (but not the same as the very slow rotation of the orbit of Mercury for instance, which Einstein explained). We could alternatively see the linear motion in "geodesists" as expression for the motion on an underlying 4-dimensional level, not belonging to a step in the same dimension chain?

2. Starting and end points of vectors as "stretched out":
The starting points of vectors inwards - - and target points of vectors outwards - have positions "stretched out", with a formulation from quantum mechanics, not defined - or all possible...
   The infinity of starting points of Direction inwards as a virtual, circular structure may be interpreted as transformed to Rotation with a factor of Time, that's Motion.
   Compare the indeterminable principle: If a particle has a certain moment, implying direction, (as inwards from the 00-pole towards a center in our model), this implies that it has all possible positions. If it has a certain position (as the 0-pole in our model), it has all possible directions. And here the 00-pole is just defined as "anticenter". Compare that the 00-pole also represents multiplicity in our model.
   In a surface, the 3rd d-degree is indefinable, and in 3rd d-degree the 4th d-degree should be likewise indefinable. The higher d-degrees have the character of "superpositions" (sooner "sub"-positions).
   Rotation, illustrating the "meeting points" between targets of outward direction and starting points of inward directions, may be regarded as expression for the binding force between mass and empty space (E= +mc2/E=-mc2) - ultimately an expression for d-degree 5 as the binding force between center and anticenter. This in accordance with our description of d-degree 5 as step by step translated into motion through the dimension chain.
   Hence, rotation in d-degree 3 should be interpreted as resulting from the combination of a radial and circular geometry.

As to a higher d-degree as indefinable in rotation, a similar description is given for quantum numbers of electron shells in the atom: two of the quantum numbers (s l, x) are fixed, defined, but the 3rd not: it is illustrated as a vector with fix starting point but the arrow of which rotates around the y-axis.
   (The slow rotation of the "y-axis" of the Earth seems to illustrate the same?)

3. How do physicists and astronomers explain Rotation?
The fact is that they don't seem to have any common explanation. One vague suggestion is that rotation should result from some irregularities in the surrounding gravitational field. If so, we could compare with the general assumption in our model that the 00-pole as anticenter, ~ surroundings, represent the polarizing force. Here in the step 4 →3.
   Other sources refer more accurately to the law of energy preservation: When a celestial cloud contracts through gravitation, the potential energy of the outer areas in the cloud decreases and the energy has to transform into something else: rotation. To a certain degree it may transform to temperature radiation outwards until the density becomes too high. There is a change in the "quality" of energy (a formulation attributed to Sarfatti).
   If this view is an explanation or just a description may be discussed. In any case it is in accordance with the general proposal in our model that a) there is a geometrical transformation from outwards-inwards to a radial - circular one, b) it is in a certain sense the inward direction from the anticenter that transforms into a circular geometry.

One author talks about "random motions which probably show a little surplus in one direction" and with decreasing radius develop to rotation in that direction. A rotation of a star 17 times per second (!) is attributed to its collapse to about 20 km radius, and to the law about preservation of angular momentum.
   Another formulation: the temperature ought to increase when a celestial cloud is compressed, but the clouds of gas have an effective way to get rid of the created warmth: the energy is stored as rotation of the hydrogen atom. (Hence, not only rotation of the big clouds, even the one of individual atoms?!)

Compare the perpendicular relation between energy forms of Frequency and Amplitude in electron shells (file EM-waves): absorbed energy expressed in amplitude of electrons, outward transmitted energy as "radial" radiation, expressed in frequency.

4. Turbulence:
A corresponding transition from linear to 2-dimensional, rotational motion appears in the emergence of turbulence in gases and liquids. Why has turbulence been such a mystery for the scientists? (One of Heisenberg's questions to God: Why turbulence?)
   How does a more or less rectilinear current suddenly change to whirls and big whirls breed smaller whirls etc.?
   We could try the assumption that more substance (as one form of energy) is poured into a water current for instance than the outlet permits, in accordance with the description of celestial clouds above, which should imply that the surplus of energy had to be translated into rotation.
   According to chaos research however, this cannot explain why currents, like the Golf stream as an example, here and there begin to wind and generate whirls or debranched circular currents. Or the behavior of smoke from a cigarette. We could probably add: the Rossby waves from the jet stream of wind around the arctic pole, from which more or less circular high-pressure and low-pressure cells are debranched.
   Could we assume that everything in the way of a linear current, invisible perhaps, that can get the role of a center, curves the linear motion as if activating one pole of d-degree 4 and step 4 →3? Perhaps a reality in some water streams but not a satisfying assumption according to other examples.

Looking at our elementary dimension chain we have:

D-degree of Structure:    5 → 4 → 3 → 2 → 1→ 0/00
D-degree of Motion:    0/00    1      2      3      4       5

Chemically, solid, liquid and gas phases have in another part of this booklet series been characterized as 3-, 2- and 1-dimensional phases respectively on that higher, chemical level. (Water for instance has molecules with a plane form.)
   If our simple scheme above is possible to apply to that level, we should find a 3-dimensional motion in liquids and a 4-dimensional motion in gases: perhaps in the form of spiraling (= translation + rotation) - with addition of a motion inwards - outwards as expression for the 4th d-degree. (Inwards?!. Do we eventually find such inward directed but perhaps disregarded motions in gases?)
   There are interesting experiments in the literature showing how 3-dimensional motions are appearing in liquids (mentioned in a book about chaos):
   A spot in a liquid was observed to twirl east - west, up - down, inward - outward. And Theodore Schwenk who studied currents in a watercourse found secondary streams moving as in spirals, as one surface rolling around another. Another example: studying liquid helium, there was observed first the formation of two rotating cylinders of the liquid, then, a bit later, also waves along these cylinders. Hence, a 3-dimensional motion.
   Scientists also use the term "phase transitions" when talking about such examples of turbulence, which in our model should represent dimension steps.

The motion of a stream may represent a 4-dimensional "vector field" as it appears on that higher level of Matter. "Direction" as structure. A liquid as a watercourse - and a gas - has of course in its entirety also a mass and a surface to the surrounding, possibly developing to internal 2-dimensional "layers", and extensions as principally 1-dimensional. (The step from linear motion to rotational (2<--1), corresponds in our dimension chain to the step from the property Direction to volume, Mass, step 4→3→)
   Hence, a stepwise increasing complexity in the motional patterns could perhaps be reasonable from this point of view, as expressions for how these different properties of the stream manifest themselves?

Assuming such phase transitions, should we think of them as endogenous or not? What causes the transitions?
   One answer, in terms of abstract, general postulates in our model, is that a unit whatever it is, here a stream, always has a surrounding, corresponding to the anticenter pole 00, representing the polarizing force. Compare the talk about "external disturbances".

More concrete, a liquid has its borders, a cloud its emptier surrounding. We could imagine that the difference at the border - as defining a border in itself - is enough to represent a polarization. Difference in velocities at the borders, through friction perhaps, should be enough to create polarities.
   The different properties in structure of Direction and Mass (4 →3) seem to manifest themselves stepwise, and this could depend on a change in velocity. Velocity has in our model tentatively been identified with the dimension steps as such, representing the debranched d-degree, fundamentally expressed in the last step 1 → 0/00.

About the splitting up of whirls to smaller and smaller ones:
In a rigid body or a big whirl all parts or particles in the whirl rotate with the same angle frequency, transverse the same angle (as an area, 2-dimensional) in the same time.
   To get the same velocity, to pass the same distance (1-dimensional), the rigid body will have to crack, the big whirl split in all kinds of angle frequencies like frequencies in white noise. In this respect turbulence represents a qualitative, geometrical step from d-degree 2 →1 in motional structure repeated. (Cf. perhaps Lev D. Landau who has seen the turbulence as result of "competing frequencies", mentioned in a book about Chaos.)
   It also implies a step from rotation concerning the radius to rotation concerning the circumference, poles 3b to 3a, (from radial to circular) as a repeated "pole exchange".

To III-IV: Geometrical aspects
                Multiplicity of Mass and its Distribution